Zero Of F According To Its Multiplicity
Zero Of F According To Its Multiplicity. Other times the graph will touch the x-axis and bounce off. Identify zeros of polynomial functions with even and odd multiplicity.
I noticed the roots are only listed as distinct roots, i.e., they are not repeated according to the multiplicities.
Explains how graphs just "kiss" the x-axis where A zero has a "multiplicity", which refers to the number of times that its associated factor appears in the polynomial.
A polynomial function has a root of -6 with multiplicity 1 ...
[Solved] = 1 = 2 3 - 4 5 =6 Suppose that the polynomial ...
📈How does the multiplicity of a zero affect the graph of ...
Find Zeros of the Polynomial Function f(x)=x^3+4x^2-16x-64 ...
Find the zeros of the polynomial f(x) =4√3x²+5x-2√3 verify ...
[Solved] = 1 = 2 3 - 4 5 =6 Suppose that the polynomial ...
SOLVED:Determine the multiplicities of the real z…
Question 9 of 10 (1 point) Question Attempt: 1 of 3 ...
Solved: For The Polynomial Function Below: A. List Each Re ...
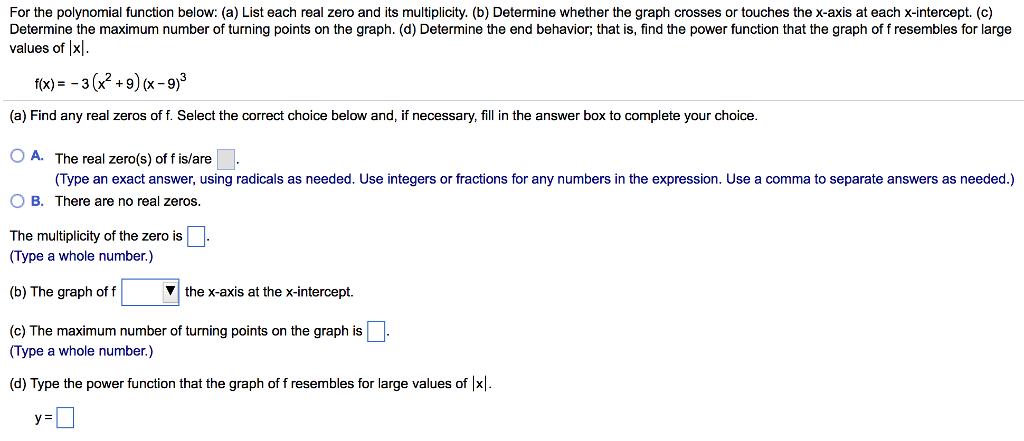
Multiplicity of Roots (or Zeros): We just saw that the real roots (zeros) of a polynomial correspond with the x-intercepts of the polynomial graph. Other times the graph will touch the x-axis and bounce off. Zero(s) of multiplicity one: None Zero(s) of multiplicity two: Zero(s) of multiplicity three Image Transcriptionclose.
0 Response to "Zero Of F According To Its Multiplicity"
Posting Komentar